Motion on Rough Inclined Plane

Block $A$ of mass $m$ and block $B$ of mass $2m$ are placed on a fixed triangular wedge by means of a massless inextensible string and a frictionless pulley as shown in figure. The wedge is inclined at $45°$ to the horizontal on both sides. The coefficient of friction between block $A$ and the wedge is $\frac{2}{3}$ and that between block $B$ and the wedge is $\frac{1}{3}$. If the system of $A$ and $B$ is released from rest, find the acceleration of $A \& B$.

The upper half of an inclined plane with inclination $\mathrm{\varphi }$ is perfectly smooth while the lower half is rough. A body starting from rest at the top will again come to rest at the bottom if the coefficient of friction for the lower half is

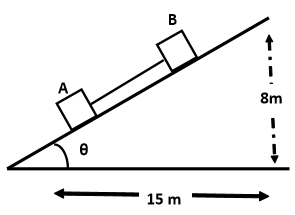
Blocks $A$ and $B$ shown in the figure are connected with a bar of negligible weight. $A$ and $B$ each has mass $170 \mathrm{kg}$, the coefficient of friction between $A$ and the plane is $0.2$ and that between $B$ and the plane is$0.4$. What is the total force of friction between the blocks and the plane $\left(g=10\mathrm{ }\mathrm{m}{\mathrm{s}}^{-2}\right)$

A body slides down an inclined plane of inclination $\theta$. The coefficient of friction down the plane varies in direct proportion to the distance moved down the plane $({\mu }_s=k.x)$. The body will move down the plane with

A body is moving down a long inclined plane of $1$ in $2$. The coefficient of kinetic friction between the body and the plane varies as $\mu =0.49 x$ where $x$ is the distance moved down the plane. The body will have its maximum velocity when it reaches:

In the figure shown, if friction coefficient of blocks $1$ and $2$ with an inclined plane is $\mathit{\text{μ}}=0.5$ and $0.4$, respectively, then find the correct statement.

A block of base $10 \mathrm{cm}\times 10 \mathrm{cm}$ and height $15 \mathrm{cm}$ is kept on an inclined plane. The coefficient of friction between them is $\sqrt{3}$. The inclination $\mathrm{\theta }$ of this inclined plane from the horizontal plane is gradually increased from $0°$. Then 

The minimum force required to start pushing a body up a rough (frictional coefficient $\mu$ ) inclined plane is $F_1$ while the minimum force needed to prevent it from sliding down, is $F_2.$ If the inclined plane makes an angle $\theta$ with the horizontal such that $\tan \theta =2\mu$, then the ratio $\frac{F_1}{F_2}$ is

A small block slides without friction down on a inclined plane starting from rest . Let $S_n$ be the distance travelled from time $t=n-1$ to $t =n$ . Then $\frac{S_n}{S_{n+1}}$ is

A box is kept on an inclined plane making an angle $\theta$ from the horizontal and coefficient of friction $\mu$. If $F_1$ is the minimum force required to start pushing it above the plane and $F_2$ to prevent it from sliding. Find the ratio $\frac{F_1}{F_2}$ if $\mathrm{tan\theta }=2\mu$.

A box of mass $6 kg$ rests upon an inclined plane. The inclination of the plane to the horizontal direction is gradually increased. It is found that when the slope of the plane is $2$ in $3$, the box starts sliding down the plane. Find the co-efficient of friction between the box and the plane. What force applied to the box parallel to the plane will make it move up the plane ? $g = 9.8 m{s}^{-2}$.

Two blocks $\mathrm{A}$ and $\mathrm{B}$ of equal masses are released from an inclined plane of inclination $45°$ at $t=0$. Both the blocks are initially at rest. The coefficient of kinetic friction between the block $\mathrm{A}$ and the inclined plane is $0.2$ while it is $0.3$ for block $\mathrm{B}$. Initially the block $\mathrm{A}$ is $\sqrt{2} \mathrm{m}$ behind the block $\mathrm{B}$. At what time in seconds will their front faces come in a line, (Take $g=10 \mathrm{m} {\mathrm{s}}^{-2}$)

A block of mass $m$ is placed on a rough plane with coefficient of friction ${\mu }_0=\frac{1}{\sqrt{3}}$ whose angle of inclination with the horizontal can be varied slowly as $0\le \theta \le \frac{\mathrm{\pi }}{2}$. If the block is tied with an elastic string of stiffness $K$ and $x$ be the instantaneous deformation in the string, then the correct variation of $x$ versus $\theta$ is given by

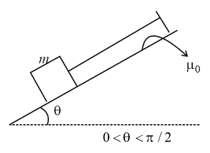
$\mathrm{A}$ particle of mass $1 \mathrm{kg}$ carrying $\mathrm{a}$ charge of $0.01 \mathrm{C}$ is able to remain at rest on $\mathrm{a}$ rough inclined plane of inclination $30°$ when a uniform horizontal electric field of $\frac{490}{\sqrt{3}} {\mathrm{Vm}}^{-1}$ is applied. Coefficient of friction is (Acceleration due to gravity $=9.8 \mathrm{m} {\mathrm{s}}^{-2}$)

A block of mass $2 \mathrm{kg}$ rests on a rough inclined plane making an angle of $30°$ with the horizontal. The coefficient of static friction between the block and the plane is $0.7.$ The frictional force on the block is

(Assume $\mathrm{g}=10 \mathrm{m} {\mathrm{s}}^{-2}$)

A block of mass $m$ is sliding down on a rough fixed inclined plane having coefficient of friction $(\mu =0.5)$ as shown in the figure. The acceleration of the block is (Take $\left.g=10 \mathrm{m}/{\mathrm{s}}^2\right)$

The upper half of an inclined plane of inclination $\theta$ is perfectly smooth while lower half is rough. A block starting from rest at the top of the plane will again come to rest at the bottom, if the coefficient of friction between the block and lower half of the plane is given by

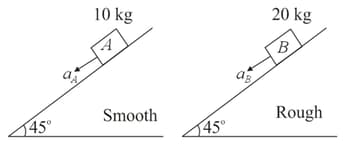
The ratio of acceleration of blocks $A$ placed on smooth incline with block $B$ placed on rough incline is $2 : 1$. The coefficient of kinetic friction between block $B$ and incline is :

A block slides down an inclined plane with an acceleration $\mathrm{g}/2$ as shown in fig. Then coefficient of kinetic friction is :-